STAAD Commands and Input Instructions
Section 5
5-142
However they are determined, the
α and β terms are entered in the
CALC data above. For this example calculate the damping ratio at
other frequencies to see the variation in damping versus frequency.
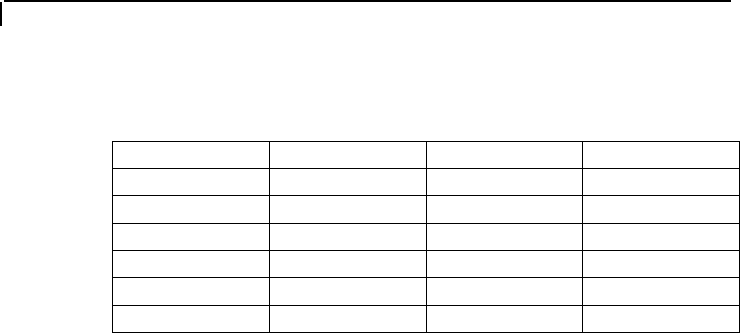
Mode Hz Rad/sec Damp Ratio
1 4.0 25.133 .04000
3 12.0 75.398 .06000
2 12.0664 .05375
8 50.2655 .04650
20 120.664 .09200
4.5 28.274 .03969
The damping, due to
β times stiffness, increases linearly with
frequency; and the damping, due to alpha times mass, decreases
parabolicly. The combination of the two is hyperbolic.
______________________________________________________
The formula used for EVALUATE (to evaluate the damping per
modal frequency) is:
Damping for the first 2 modes is set to dmin from input.
Damping for modes i = 3 to N given dmin and the first two
frequencies ω
1
and ω
2
and the ith modal frequency ω
i
.
A
1
= dmin / (ω
1
+ ω
2
)
A
0
= A
1
* ω
1
* ω
2
D(i) = (A
0
/ ω
i
) + (A
1
* ω
i
) damping for modes i = 3 to N.
If the resulting damping is greater than the dmax value of
maximum damping, then dmax will be used.
Example: (for dmin = .02 , dmax = .12 and the ω
i
given below)
Mode ω
i
Damping ratio
1 3 .0200
2 4 .0200
3 6 .0228568
N 100 .1200
(calculated as .28605 then reset to maximum entered)


















